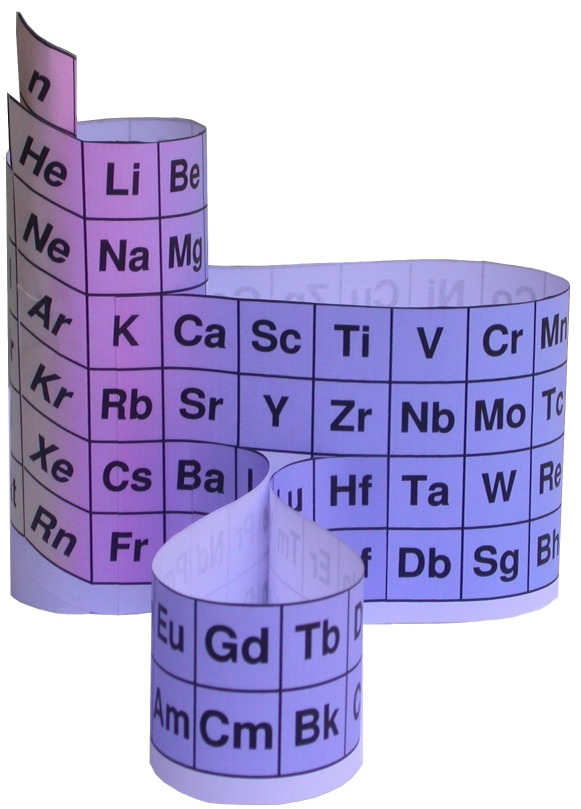
Figure 1: Three Dimensional Periodic Table, View 0
The main purpose of any periodic table of the elements is to help us understand the relationships among the various elements. We want to construct a “periodic table” that is a reasonably faithful representation of the observed relationships.
We shall see that there are many advantages to thinking of the periodic table as a three-dimensional object (rather than as a flat, two-dimensional object). The idea of a 3D periodic table is not new: in 1862 Alexandre Beguyer de Chancourtois published a description of his method for arranging the elements in a helix, on the surface of a cylinder. Note that this is several years before Mendeleev got into the game. For a good discussion of the history, see reference 1.
Devising such a table is akin to cartography. If we take something non-flat, such as the surface of the earth, and try to portray it on a flat piece of paper, there will be distortions. The cartographer’s task is to minimize the impact of these distortions, to prevent the distortions from causing too many misconceptions.
Here are several views of a 3D periodic table. The rest of this document is devoted to how it was constructed, and why.
I will explain my three-dimensional periodic table in four steps, using four figures. Figure 5 logically comes first, but for pedagogical reasons let’s skip it for now, and deal with in in section 1.5.
Figure 6 contains the “main” part of the periodic table. If you cut out the figure, you can easily roll it into a cylinder. I arranged for each row of the table to run slightly diagonally, so as to exhibit the periodicity more clearly. In particular, sodium is connected to the right of neon just as clearly as fluorine is connected to the left of neon. This part of the table is periodic, with a period of eight.
To a fair approximation you could say that the actual elements exhibit the symmetry of a cylinder, but an even better approximation would be to say that they exhibit the symmetry of a helix that winds around the cylinder.
For some discussion of the strengths and weaknesses of this table, see section 3. A straw-man alternative is discussed in section 7, and a better alternative is discussed in section 8.
There is a conspicuous gap under aluminum in figure 6. The elements that fill this gap include the transition metals, shown in black in figure 7. You could cut out this figure and paste it onto the previous figure, forming a big bulge on the side of the cylinder.1 Note that both scandium and gallium have an equal claim to sit “directly” under aluminum. (The set of transition metals does not include the elements shown in gray in figure figure 7. Those are there just for context.)
The top row of transition metals consists of 11 elements, namely 21Sc to 31Ga inclusive. Loosely speaking, we can envision these 11 elements as being eka-aluminum plus some number of d-electrons, where the number runs from 0 to 10 inclusive. (This way of envisioning things is partly consistent with the data and partly not, as discussed in section 3.6.)
There is a conspicuous gap under yttrium in figure 7. The elements that fill this gap are the lanthanoids, as shown in black in the top row of figure 8. The name “lanthanoids” applies to 15 elements, namely 57La through 71Lu inclusive. (It does not apply to the elements shown in gray in figure 8. Those are just there for context.) On the row below the lanthanoids are the actinoids.
You could cut out this figure and paste it onto the previous structure, forming a bulge on the side of the previous bulge. Note that both lanthanum and lutetium have an equal claim to sit “directly” under yttrium. To say the same thing another way, we can envision the lanthanoids as being eka-yttrium plus some number of f-electrons, where the number ranges from 0 to 14 inclusive. See section 3.6 for more on this.
So we have a bulge on the side of a bulge. That is, the lanthanoids and actinoids form a bulge on the side of the transition metals, just as the transition metals form a bulge on the side of the “main” part of the periodic table. The transition-metal bulge is associated with the belated filling of a d-electron subshell, while the lanthanoid/actinoid bulge is associated with the belated filling of an f-electron subshell.
See also section 3.3 and section 3.5.
As promised, we now return to discussing figure 5.
In figure 5, the symbol n stands for neutron. (Note that uppercase N represents nitrogen, while lowercase n represents neutron. Similarly, lowercase e represents electron, and lowercase p represents proton.)
A neutron has atomic number Z=0 (no protons), just as hydrogen has atomic number Z=1 (one proton), and helium has atomic number Z=2 (two protons). The periodic table arranges things in order of atomic number, so the sequence n, H, He is perfectly consistent with the overall pattern.
On the other hand, it is true that n isn’t an element, so one could argue that it doesn’t belong anywhere on a periodic table of the elements. Still, it’s harmless where it is, and it serves the practical purpose of giving the model a good place to attach the left side of the hydrogen box.
The chemistry of hydrogen is exceptional. There’s no getting around that. Any attempt to fit hydrogen in to one of the 8 columns in the main part of the periodic table would be sheer lunacy.
Whereas the main part of the periodic table has a period of 8, as shown in figure 6, the hydrogen row has a period of only 2, and cannot be fitted into figure 6 satisfactorily. So logically what we should do is cut out figure 5 and roll it into a tall thin cylinder, with a periodicity of 2. Hydrogen is the only non-noble-gas element in this tall thin periodic table.
Logically, the next step is to paste figure 6 onto the aforementioned tall thin cylinder, so as to cover up the gap below hydrogen. This is logical ... but it is awkward, because the bulge is so much bigger than the cylinder it is attached to. On the other hand, this way of looking at things emphasizes the noble-gas column as the anchor and backbone of the whole periodic table, which it is.
So, given a periodic table with a gap beneath hydrogen, we then plug in figure 6 to fill in the gap.
Some people claim lithium should be placed directly below hydrogen. This makes a certain amount of sense, because hydrogen commonly exhibits a valence of +1. At the other extreme, some people claim that fluorine should be placed directly below hydrogen. Again this makes a certain amount of sense, because hydrogen commonly exhibits a valence of -1 ... to the extent that valence means anything. Meanwhile, some people claim carbon should sit directly below hydrogen, since it has a similar electronegativity.
Arranging the periodic table as a cylinder with bulges allows you to satisfy all three claims simultaneously, if you want to. That is, you could say that lithium and fluorine and carbon are all claimants to the eka-hydrogen role.
The problem is, none of those claims fit the facts very well. That is, no element has a very good claim to the eka-hydrogen slot in figure 5. This situation is not even remotely similar to the lanthanoid situation, which can be rather well described as 15 elements all of which have a good claim on the eka-yttrium slot.
We need to look at the physics, rather than guessing, or wishing, or playing numerological games. The fact is that the H+ ion is very small. It’s just a bare proton. It’s about five orders of magnitude smaller than the Li+ ion. So if you describe LiF as a Li+ ion next to an F+ ion, you ought not describe HF in the same terms. It would be better to describe HF as a H+ ion deep inside the F+ ion.
From this point of view, it seems quite pointless to argue about the valence of hydrogen or the electronegativity of hydrogen. The hydrogen atom is going to do what it does ... and many of the things it does are not analogous to what any other atom does.
So, when we paste figure 6 into the gap in figure 5, the question arises of which elements “line up” below hydrogen. The question, alas, is not really answerable. The closest I can come to an answer is to say, very informally, that hydrogen is about 10% lithium-like, 10% carbon-like, 10% fluorine-like, 10% all of the above, and 60% none of the above.
Practical hint: By way of strain relief, you should trim the connection between He and Ne. Cut the left 1/3rd of the width and keep the right 2/3rds of the width. This allows the left 1/3rd to participate in the high curvature of the N=1 period. Similarly you should cut the right 1/3rd of the connection between n and He. In both cases, the cut is on the side nearest H, and the purpose is to lessen the workload on the H box. If you don’t do this, the N=1 “cylinder” will be squashed flat.
Some people are fond of drawing what they call a “full” periodic table (also known as an “extended” periodic table). Here is one good way of doing it:
Let me point out some features of this table. We can continue to think of it as a cylinder. It has periodic boundary conditions; for example the neon on the in the rightmost column is the same in every way as the neon in the leftmost column.
A cylinder without bulges could be slit and unrolled to make a smooth, flat chart. In contrast, if there are bulges, bad things happen when you try to flatten it. The elements directly above the bulge will get ripped in half. In figure 9 you can see that boron and aluminum have been ripped in half to “make room” for the transition metals, while scandium and yttrium have been ripped in half to “make room” for the lanthanoids and actinoids.
When there are two equally-good places to put an element, I put it in both places. Take yttrium for example: it makes equally good sense to line it up with the first of the lanthanoids (La) and/or to line it up with the last of the lanthanoids (Lu) … so I do it both ways.
You can compare and contrast this with the corresponding table featured in reference 2.
We start by pointing out some nice features of the “main” part of the periodic table, as shown in figure 6 and preserved in figure 9:
On the other hand, putting tin and lead in the same column as carbon and silicon is an example of letting the naïvely theoretical tail wag the factual dog – although I haven’t seen any good way of improving this. My point is that finding an element in a particular column of the periodic table sometimes predicts its properties, and sometimes doesn’t. As we go around the cylinder in either direction, columns near noble-gas column are more well-behaved than columns farther away.
A more subtle problem is that based on the observed chemical behavior, we might want to treat Zn the same way as Ca, and treat Ga the same way as Sc ... but the color code in these tables does not attempt to represent the chemistry. Instead, the color-code just indicates what column the element is in. The color-code depends on the atomic number Z, and not much else. That is, the color changes monotonically as a function of Z as we go across each row. It is not meant to encode any particular chemical property.
Any attempt to construct a periodic table is beset by a dilemma:
The problem is, the physical properties of the elements do not vary monotonically as a function of atomic number. So we satisfy the requirements as best we can, making compromises where necessary.
In other versions of the periodic table, in the second and third rows, one often sees a big gap between beryllium and boron, and between magnesium and aluminum. This gap exists solely to make room for the transition metals. The gap is misleading as to the Be/B relationship and the Mg/Al relationship.
To understand what’s going on, let’s look at the fourth row and consider the 11-member series from scandium to gallium inclusive. Let’s see how the end-members (Sc and Ga) fit relative to the previous row, and also examine the context, i.e. the nearest neighbors to the right and left. Here are the typical oxides:
| Valence: | 2 | 3 | 4 | |||||||
| Row 3: | MgO | Al2O3 | SiO2 | |||||||
| Early Row 4: | CaO | Sc2O3 | TiO2 | <OTE> | ||||||
| Late Row 4: | <OTE> | ZnO | Ga2O3 | GeO2 |
where the transition elements are in boldface, and <OTE> indicates Other Transition Elements. The table shows the common, typical oxides, but not all possible oxides. In particular, peroxides are often possible, but they are much less common.
Here are the unadorned elements:
| Valence: | 2 | 3 | 4 | |||||||
| Row 3: | Mg | Al | Si | |||||||
| Early Row 4: | Ca | Sc | Ti | <OTE> | ||||||
| Late Row 4: | <OTE> | Zn | Ga | Ge |
The early row 4 chemistry suggests we should put Sc under Al, and put transition elements (at least some of them) to the right of there. This is not the traditional arrangement, but it makes as much sense as anything else.
Meanwhile, the late row 4 chemistry suggests we should put Ga under Al, and put transition elements (at least some of them) to the left of there. This is more-or-less the traditional arrangement.
So far we have accounted for 4 of the 11-member series: the end members (Sc and Ga) and the next-to-end members (Ti and Zn).
Deeper in the interior of the sequence, things get messy. There are four possible chromium oxides, including CrO3, CrO2, Cr2O3, and Cr3O4. There are five possible oxides for manganese. This means transition elements can participate in interesting redox reactions, but it also means they don’t fit into any of the colums defined by the elements in the “main group” i.e. rows 2 and 3. They don’t even fit well with each other; in the cell below Cr, there are ordinarily three oxides for molybdenum.
For constructing our 3D table, we don’t need to lose too much sleep over this mess. We can make sense of things locally, as shown in table 2. The fact remains that both scandium and gallium act like eka-aluminum, and their nearest neighbors to the left and right behave as expected. This suffices to tell us where the transition-metal group belongs: the entire 11-member group, from 21Sc through 31Ga inclusive, belongs under aluminum. This is not a perfect fit, but there is no better place. Putting all the transition elements to the left of eka-aluminum is not uniformly better than putting them to the right. Similarly, the widespread practice (e.g. reference 3) of putting gallium (but not scandium) directly under aluminum is by no means the best way of representing the observed properties.
As for terminology, there are multiple ways to spin it: The term “transition elements” could reasonably be applied to the full 11-member series (Sc through Ga inclusive) or to the 9-member series (Ti through Zn inclusive). It would be less reasonable to construct a 10-member series by including one of the trivalent end-members and not the other (Sc or Ga but not both). Alas, even though the 10-member approach is the least reasonable, it is the most prevalent.
The purpose of the periodic table is to summarize and predict the properties of real elements. Many of the predictions are good ... but none of them are exact. We would not expect them to be exact, since the details of atomic structure are fantastically complicated. The details cannot possibly be fully represented by any two- or three-dimensional chart.
There are 15 lanthanoids, from 57La through 71Lu inclusive. This grouping is very well supported by the observed chemical properties. As a rule (with minor exceptions) the lanthanoids all act like each other, and all act like eka-yttrium, so it makes sense to place all 15 lanthanoids in the periodic table directly under yttrium.
The rule is particularly clear when we look at the end-members of the series, and compare them to their neighbors: Lanthanum is chemically much more like the other lanthanoids than it is like barium. At the other end, lutetium is much more like the other lanthanoids than it is like hafnium.
More generally, the 15 lanthanoids tend to form halides as LCl3 and oxides as L2O3 (where L stands for any lanthanoid). This is in analogy to YCl3 and Y2O3, and in contrast to BaCl2, BaO, HfCl4, and HfO2. You should check the data for yourself; see for example reference 3.
Virtually all our observations about lanthanoids apply equally well to the 15 actinoids, namely 89Ac to 103Lr inclusive.
The similarity of one lanthanoid with another is even more pronounced than the similarity of one transition metal with another. Therefore figure 8 does an even better job of representing the real world than figure 7 does.
See also section 1.3 and section 3.5.
The two mighty powers have, as I was going to tell you, been engaged in a most obstinate war for six-and-thirty moons past. It began upon the following occasion. It is allowed on all hands, that the primitive way of writing the periodic table was to put La below Y, but the emperor published an edict, commanding all his subjects, upon great penalties, to put Lu below Y. The people so highly resented this law, that our histories tell us, there have been six rebellions raised on that account; wherein one emperor lost his life, and another his crown. These civil commotions were constantly fomented by the monarchs of Blefuscu; and when they were quelled, the exiles always fled for refuge to that empire. It is computed that eleven thousand persons have at several times suffered death, rather than submit to anchor their lanthanoids at the smaller end. Many hundred large volumes have been published upon this controversy: but the books of the Big-endians have been long forbidden, and the whole party rendered incapable by law of holding employments. During the course of these troubles, the emperors of Blefuscu did frequently expostulate by their ambassadors, accusing us of making a schism in religion ......
Compare reference 4.
Consider the contrast:
|
|
For reasons discussed in section 3.3, by far the best approach is to put all 15 lanthanoids in the eka-yttrium slot. This treats all lanthanoids on the same footing, all 15 of them, from La through Lu inclusive. There are at least three ways of doing this:
See also section 1.3 and section 3.3.
If you look up2 the ground-state electron configuration (the “GSeC”) of each element in the first row of transition metals – 21Sc to 31Ga inclusive – you find that the GSeC is not well described by the pattern tentatively suggested in section 1, namely “eka-aluminum plus some number of d-electrons”.
That’s a small problem for us, but not a huge problem. The GSeC pertains to the physics of the isolated atom, whereas for present purposes we care more about the ordinary chemistry, i.e. how the behaves inside a molecule. The periodic table suggests a pattern, and the actual, practical chemistry of scandium fits this pattern better than the GSeC does. If we were fixated on the ground state of the isolated atoms, we might be worried that the Al valence shell is s2 p1 whereas the Sc valence shell is s2 d1 (for the ground state of the isolated atom). But we are not fixated, so we are not going to worry about that. We’re not going to ignore the GSeC completely, but we’re not going to overemphasize it, either.
It is a mistake to think of the GSeC as «the» electron configuration. The fact that the GSeC is commonly tabulated on periodic tables may be part of the reason why some people overestimate its importance. This is quite a common mistake, but it is still a mistake.
When we come the lanthanoids and actinoids, the small problem is even smaller. To a good approximation, the chemistry of the lanthanoids is what we would expect for eka-yttrium. As for the ground-state electron configuration, the lanthanoids collectively can be described approximately (not exactly) as eka-yttrium plus some number of f-electrons. This is particularly clear for the end-members of the lanthanoid series, both of which look like eka-yttrium:
| Y | : | [Kr] | 4d1 | 5s2 | |
| La | : | [Xe] | 4f0 | 5d1 | 6s2 |
| Lu | : | [Xe] | 4f14 | 5d1 | 6s2 |
In the interior of the lanthanoid series, the GSeC numbers are not quite so simple. However, we again emphasize that for most purposes, the way the elements behave in chemical reactions is more important than the ground-state electron configuration of the isolated atoms. The lanthanoids all behave pretty much the same, even more the same than their GSeC numbers would suggest.
Consider the assertion that the chemistry of Zn is similar to the chemistry of Ca.
Reference 3 is a convenient source of data that you can use to check such assertions. On each element’s main page, over on the right side, there is a box entitled “compounds”. On the calcium page we learn that its fluoride is CaF2 and its oxides include both CaO and CaO2. Similarly on the zinc page we learn that its fluoride is ZnF2 and its oxides include both ZnO and ZnO2. And that’s not all; for each element, there are about 10 compounds listed; you can check for yourself.
Good science does not depend on taking somebody’s word for something.
You are not required to check every assertion that you encounter, but every assertion should be sufficiently well documented that you could check it if you wanted to.
We can deepen our understanding of the periodic table by using some simple ideas from atomic physics. One essential idea is that a completely full shell is almost as nonreactive as a completely empty shell. (This is a well-known rule, sometimes listed as one of Hund’s rules; see reference 5.)
We have to be slightly cagey about what we consider a “shell”. There is a difference between mathematics and physics. Mathematics will tell you the symmetry of the Ylm spherical harmonics, but will not tell you the energy of the atomic energy levels.
Yes, closing out the N=1 shell gives us helium, which is the first noble gas. And yes, closing out the N=2 shell gives us neon, which is the second noble gas. However, the third noble gas, argon, does not (in the mathematical sense) close out the N=3 shell, because the 3d orbitals remain empty. So we see that chemical idea of closed third shell does not correspond to the mathematical idea of filling up all the N=3 orbitals. The distinction depends on the energy; the neon 3d orbitals are more-or-less inaccessible because they are too high in energy.
Another essential idea is that chemical properties are determined by the valence electrons, while the non-valence electrons don’t matter very much. This is an approximation, but it is often a good approximation. For example, potassium and sodium have the same valence-electron configuration, and differ only by a closed shell of non-valence electrons. This leads us to expect them to be chemically very similar, as indeed they are. To a fair approximation, potassium is just heavier.
If potassium didn’t already have a name, we could perfectly well call it eka-sodium (following a naming convention that goes back to Mendeleev).
Caveat: Although talking about «the» valence of a given element makes sense at certain locations in the periodic table (notably the noble gases and the alkali metals), at other locations (notably the transition metals) we need to be more careful. For instance, we know that copper can form both cupric chloride (CuCl2) and cuprous chloride (CuCl).It is traditional for periodic tables to display «the» electronic configuration for each element. If you read the fine print, it says this is the electronic configuration for the ground state of the isolated atom. As discussed in section 3.6, the GSeC is sometimes not useful, and sometimes very misleading. You are very unlikely to encounter isolated ground-state copper atoms in the chemistry laboratory. The copper atoms you do encounter will be ionized and/or bonded to other atoms. The ionic and molecular electronic configuration is determined by many factors, not solely by the atomic ground state.
The f-electrons almost never participate directly in covalent bonds. They don’t stick out far enough, not nearly as far as the s and p electrons do.
I don’t know any simple atomic-physics explanation of why the lanthanoids sit exactly where they do in the periodic table. They have to sit somewhere of course, but it would be a tour de force to prove from first principles why they act like eka-yttrium as opposed to (say) eka-strontium.
One thing is for sure: There are very strong theoretical and observational reasons why La and Lu should belong in the same column of the periodic table. There is no logical basis for putting one of them – and not the other – under Y.
A more-common but less-satisfactory version of the periodic table is shown in figure 10, using the “medium width” format.
The same idea is shown in figure 11, using the “full width” style.
The problem with both these figures is that a particular type of «block structure» has been imposed, as reflected in the labels and in the color-coding in the figures. This sort of block-structuring has some superficial advantages and some fundamental problems.
Let’s consider the following pros and cons. The pros are remarkably weak, and can be considered “damning with faint praise”.
| The blocks make the table appear to have a simple, precise, systematic structure. | The problem is that this table-on-paper does not reflect the actual properties of the chemical elements. A lesser claim of precision would fit the facts better, for the same reason that it is far better to say “π = 3.1±0.1” than to say “π = 3.1 exactly”. |
| The blocks are superficially attractive. They look nice from a distance. It is understandable that people find rectangular regularity more esthetically pleasing. | We must not let the esthetic tail wag the scientific dog. Esthetics is not science. |
| I suppose a regular rectangular pattern is easy to remember. | It is unhelpful to have an easy way of remembering «facts» that are not true. The raison d’être of the periodic table is to reflect the properties of the real chemical elements ... which the «f-block», «d-block», etc. presentation does not. |
| The numbers that specify the width of the blocks (2, 6, 10, and 14) are the same numbers we encounter in atomic physics. | Numerology is not science. See the discussion of fencepost errors, below. |
| The block-structured periodic table appears in many textbooks. | Dogma is not science. |
| When constructing a theory, everyone is free to define things however they like. | In reality, Mother Nature is under no obligation to pay attention to such definitions. |
| It is straightforward to define the term f-block. | Alas, the f-block idea doesn’t comport with physical and chemical reality. |
At the next level of detail, we should consider the following points:
All in all, it does not appear that calcium’s claim on the eka-magnesium slot is particularly stronger than zinc’s claim.
The same thing happens again on the next row: strontium’s claim to the eka-calcium slot is not particularly stronger than cadmium’s. There is no good reason why Sr and Cd should be in categorically different blocks.
The same thing happens yet again on the row below that: barium’s claim to the eka-strontium / eka-cadmium slot is not particularly stronger than mercury’s. There is no good reason why Ba and Hg should be in categorically different blocks.
The same thing happens again on the next row: yttrium’s claim on the eka-scandium slot is not particularly stronger than indium’s. There is no good reason why Y and In should be in categorically different blocks.
The same thing happens yet again on the row below that: The lanthanoids’ claim to the eka-yttrium / eka-indium slot is not particularly stronger than thallium’s.
The same thing happens on the next row: However you align the lanthanoids, you should align the actinoids the same way. There is no good reason to put Ac and Lr in categorically different blocks.
When I say two elements have “comparable claims”, that is based on solid theory (section 5) and on observation (section 4). It is easy to understand in terms of atomic physics why a completely filled shell should behave very similarly to a completely empty shell, as discussed in section 5. The theory is supported by innumerable observations, as discussed in section 4. Applying this idea to the present discussion, we find that La and Lu are the same, except that one has a completely empty 4f shell, while the other has a completely full 4f shell. Theory predicts that they should be chemically very similar, and this is in fact observed. It is hard to imagine why anyone would put them in different «blocks».
One should never support a strong argument with a weak one, but it is amusing to note that about half of the schemes collected in reference 2 put La (not Lu) below Y, and about half do it the other way. This suggests that nobody has a very convincing reason why either way is better. In my opinion, both are equally silly.
All lines of evidence suggest we should represent the periodic table as a cylinder with bulges. This has the advantage of giving La and Lu equal status as members of the bulge below Y.
I am comfortable talking about 15 lanthanoids and 15 actinoids, as illustrated in figure 8, because the concept is well supported by the data and the theory. In contrast, I have never willingly used the term «f-block elements» except in scare quotes, because the concept is not well supported by the data, nor by legitimate theory (where by ‘legitimate’ I mean to exclude numerology).
The existence of the lanthanoid series is related to the filling of
the atomic f-orbital.
– Yes, there are at most 14 f-electrons in any given atom.
– No, that does not mean there are at most 14 lanthanoids.
The square directly below yttrium in figure 7 can be called the eka-yttrium square. As discussed in more detail in section 5, the entire lanthanoid series can be fairly well described as eka-yttrium plus some number of f-electrons. The point is, when we say “some number” of f-electrons, the number runs from 0 to 14 inclusive, for a total of 15 possibilities, i.e. 15 lanthanoids.
Let’s be clear about the arithmetic: if you have items labeled 0 to 14 inclusive, that makes 15 items. This is not a chemistry issue. This is not a physics issue. This is just arithmetic. (If you get this wrong, it’s called a fencepost error. See reference 6.)
I emphasize this because many people who ought to know better believe there are only 14 lanthanoids. This is pretty astonishing, especially considering that to arrive at such a misconception you need to make at least two mistakes. That’s because the right answer is well supported by data as well as theory. So to get the wrong answer you have to ignore the data and then misunderstand the theory.
Some periodic tables, to their discredit, make this mistake quite explicit. Reference 3 labels La as an f-block element (color-coded green) and labels Lu as a d-block element (color-coded red).
In contrast, to its credit, IUPAC (reference 7) lists all 15 lanthanoids together, leaving a hole in the eka-yttrium square, as we have done in figure 7.
If you do the physics right, it tells you that there are 15 lanthanoids. They can be reasonably well described as eka-yttrium with some number of f-electrons, where the number runs from 0 to 14 inclusive.
Those who propose a 14-element «f-block» are free draw their periodic tables any way they like, and they are free to color-code their «f-block» elements any color they like. But they should not expect Mother Nature to pay any attention to what they have done.
It just cracks me up when people who don’t understand atomic physics try to claim that figure 11 is based on physics. Let’s be clear:
This stands in contrast to the correct physics, which agrees with the observed chemistry.
To get a feeling for the sort of engineering that goes into the design of a periodic table, consider the hypothetical alternative versions shown in figure 12 and figure 13. The transition elements start one element earlier and end one element earlier, compared to the version presented in section 1. Consider the comparison:
| Standard statement: 21Sc and 31Ga have an equally good claim to sit directly below aluminum. | Alternative statement: 20Ca and 30Zn have an equally good claim to sit directly below magnesium. |
Neither statement is significantly better or worse than the other.
We cannot continue this process another step in either direction. That is, nobody thinks 19K is closely analogous to 29Cu, and nobody thinks 22Ti is closely analogous to 32Ge (although it is possible to have TiF4 in analogy to GeF4).
Loosely speaking, the story that goes with figure 13 is that we can describe calcium through zinc as being eka-magnesium plus some number of d-electrons, where the number runs from 0 to 10 inclusive. This story is not nearly so precise as the corresponding story about the lanthanoids, because the transition-metal d-electrons are vastly more chemically active than the lanthanoid f-electrons. Also, this version of the story does not fit the data any better than the more conventional version based on eka-aluminum, as mentioned in connection with figure 7.
Actually, the hypothetical version is slightly disadvantageous for some purposes. For one thing, the color-coding of the lanthanoids in figure 14 wrongly suggests they are more akin to the divalent alkaline earths than they are to the often-trivalent transition metals. Partly this means that the hypothetical color coding is wrong, but it is also a hint that the hypothetical columns are not lined up optimally.
When designing a periodic table, we cannot escape the fact that the potassium row is ten elements longer than the sodium row, so we need to stick the extra elements somewhere. We should choose somewhere that does not unduly disrupt the vertical correspondences in the table (alkali metals all in the same column, halogens all in the same column, et cetera). We also need to preserve the ordering of the elements, i.e. in order of atomic number. This last requirement is tricky. It cannot be based solely on simple chemical data, because many important chemical properties – notably the valence of the transition metals – are complicated non-monotonic functions of atomic number.
Let’s combine the ideas of section 7 with section 1. In particular let’s retain both the gap under Al from figure 6 and the gap under Mg from figure 12. The result is shown in figure 15.
That leaves us with 12 columns of elements to patch into this double-width gap, as shown in figure 16. Note the column headers (2, 3) near the upper left and again near the upper right. The bulge carrying the transition metals floats above the second and third columns of the main part of the table.
According to this arrangement, the 12 elements from 20Ca through 31Ga inclusive can be described as eka-magnesium or eka-aluminum plus some number of d-electrons, where the number runs from 0 through 10 inclusive.
This description has some strengths and some weaknesses. The strengths include the following: In the first row of figure 16, starting from the left of the row we have K–Ca–Sc whose observed behavior fits nicely under Na–Mg–Al, with “valence” 1–2–3, so the table is faithful to reality in this regard. Similarly at the right end of the top row, we have Zn–Ga–Ge whose observed behavior fits nicely under Mg–Al–Si, with “valence” 2–3–4, so this is another place where the table is faithful to reality. The point is that the “light 2” and “light 3” (namely Ca and Sc) compete more-or-less equally with the “heavy 2” and “heavy 3” (namely Zn and Ga) when competing for the two slots below Mg and Al.
The weaknesses are concentrated in the middle columns of figure 16. As an extreme example, the observed chemistry of chromium is not what you would expect from eka-magnesium or eka-aluminum. Forsooth chromium does not behave like eka-anything, so any attempt at a faithful periodic table is going to have problems. This is understandable, because the d-electrons are not chemically inert. In any case, though, figure 15 makes at least as much sense as figure 6, and more sense than figure 12.
The design principle here harks back to Hippocrates: “First, do no harm.” In this case that means that when there are two comparably-good claimants to a particular spot in the table, rather than arbitrarily choosing one and hanging the other out to dry, it is better to put a patch over the contested spot, so that the patch can show both contenders on an equal footing.
The diagrams in section 10 are available in Scalable Vector Graphics (.svg) format, and also in Encapsulated PostScript (.eps). Both are modern open standards. Using a scalable format means you can shrink or enlarge the diagram as much as you want without losing any detail.
To download the scalable files, click on the [svg] link or the [eps] link at the right side of the caption.
You should then be able to read your copy of the file into any image processing program worthy of the name, rescale it, and write it back out again. The .svg format is better for embedding in HTML documents; the Firefox browser can render .svg natively. Meanwhile, the .eps format is more widely recognized by other applications.
In this section, the first three figures – namely figure 17, figure 18, and figure 19 – are periodic tables that display some basic data for each element, namely
The color coding in these three figures reflects the element’s position within the period.
Figure 20 is the same as figure 18 except that it doesn’t use color. This is useful if you have a printer that doesn’t reproduce color.
In figure 21, the bottom row in each cell shows the first ionization potential. Also, the color coding is based on the first ionization potential.
The term rare earth is also widely used. It is often used to refer to the lanthanoids by themselves, but historically it had a different meaning, namely the set consisting of scandium, yttrium, and the 15 lanthanoids (without the actinoids). See reference 8 for more about this.
They earned the name “rare earths” because of some quirks of geochemistry. Most of the naturally-occurring rare earths are spread out in low concentrations throughout the crust. The concentrations are so low that they are hard to notice, let alone exploit commercially. This stands in contrast to (say) copper, silver, and gold, which are rare in absolute terms, but are more likely to be found in commercially-important concentrations.
As for the actinoids, uranium is more abundant than tin, and thorium is even more abundant than that. The other actinoids are have very little (if any) natural abundance. This is what you would expect, based on their short half-lives.
To fully appreciate the 3D periodic table, you can build an actual three-dimensional model. You will need a pair of PDF files. There are different pairs you can choose, depending on your choice of the size of model and the amount of data to be shown.
| colored | b&w | |||||||||||||||||
| symbol only | sym, name, data | |||||||||||||||||
| A4/letter |
|
| ||||||||||||||||
| legal |
|
|
Note: Be sure to set your printer options so that the images are printed at actual size. Specifically: any “scale to fit” options must be turned off.
The general idea is to print out the files, cut out the pieces, and tape them together. I recommend using double-stick tape, because it is easy to work with and produces good results.
The typical joint is made using tape to join the back (unprinted) side of part “A” to the front (printed) side of part “B”. It is recommended to start by applying tape to the back side of part “A” – and then joining that to part “B”. That’s because you want the tape to be aligned flush with the edge of part “A”, as closely as possible. Aligning the tape with the edge of part “B” would not produce the desired result. If the pieces are already cut out, do the best you can to put the tape into place; however, a cleverer technique is to apply the tape to the backside of the paper before final trimming, so that when you trim the paper you trim the tape, making it exactly flush.
If you are using the small mandrel as a rolling pin, you may want to also have an area of slightly sticky material on the table-top, to hold one edge of the paper while you are rolling up the other edge.